
Though the reverse of the statement is not true. Whereas, rational numbers are numbers which can be expressed in the form of $\dfrac = 1.5$ that is a decimal not an integer. Integers are all whole numbers that can be marked on the number line, negative and positive numbers. Let’s finish by recapping some of the important points from this explainer.Hint: In order to make a relation between rational numbers and integers, we should first know what is rational number and what are integers. Rational numbers include all integers and fractions. Hence, only option C is a rational number. Real numbers are mainly classified into rational and irrational numbers. Which is the quotient of two integer values where the denominator is nonzero. Thus, neither of these represents rational numbers. We have a similar story in expressions B and D. Since we cannot divide by 0, this is not a rational number. We can determine which of these expressions is rational by substituting the values of 𝑎 and 𝑏 into each expression separately.ģ 9 𝑏 𝑏 − 3 4 = 3 9 ( 3 4 ) 3 4 − 3 4. We begin by recalling that the set of rational numbers, written ℚ, is the set of all quotients of integers. Which of the following expressions is rational given 𝑎 = 1 and 𝑏 = 3 4?
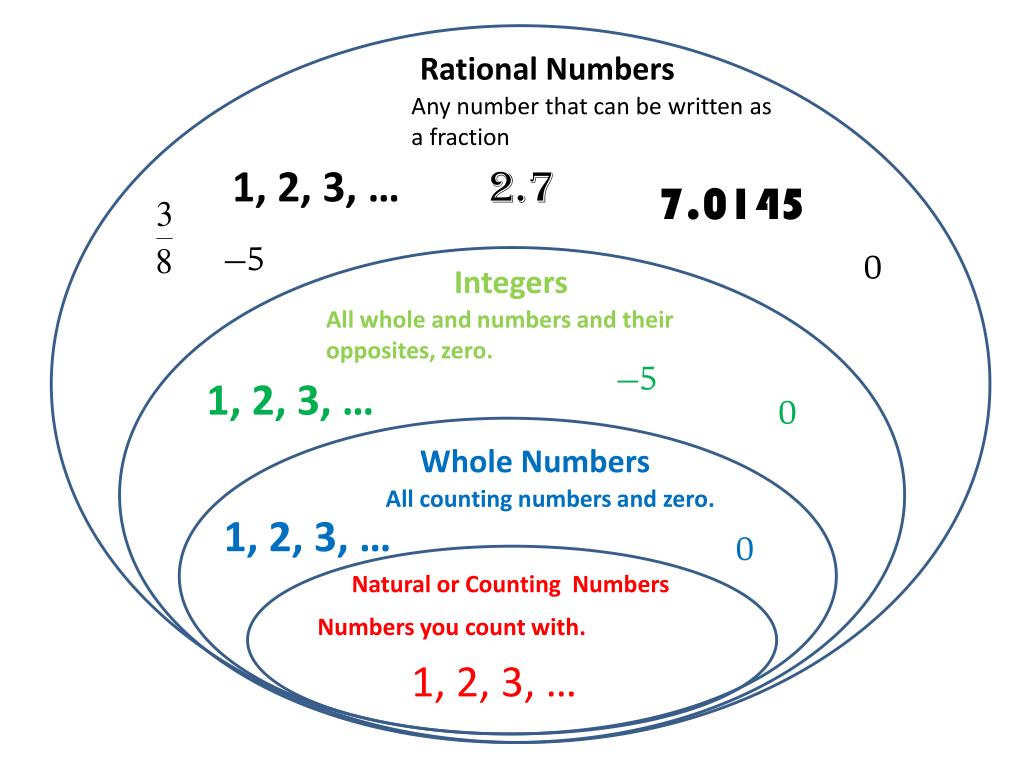
We can represent this information in the following Venn diagram.Įxample 6: Identifying the Rational Expression from a List of Given Expressions Represent fractions as mixed numbers for example, 5 3 = 1 2 3, which is also a rational number. We can represent numbers like this using a line It is worth noting that any decimal expansion with a finite number of digits or a repeating expansion is rational. 5, so we can also represent rational numbers as decimals.

This is not the only way of representing rational numbers we have also seen that 1 2 = 0. For example, we can think about 5 3 as 5 lots of 1 3. One way of conceptualizing rational numbers like these is toĬonsider them as multiples of simpler fractions. Similarly, 5 3 and 1 2 7 are rational numbers. (An integer itself has no fractional part.) Example: 1.5 is a rational number because 1. For example, 1 and 2 are integers, so 1 2 ∈ ℚ. A Rational Number can be made by dividing an integer by an integer.
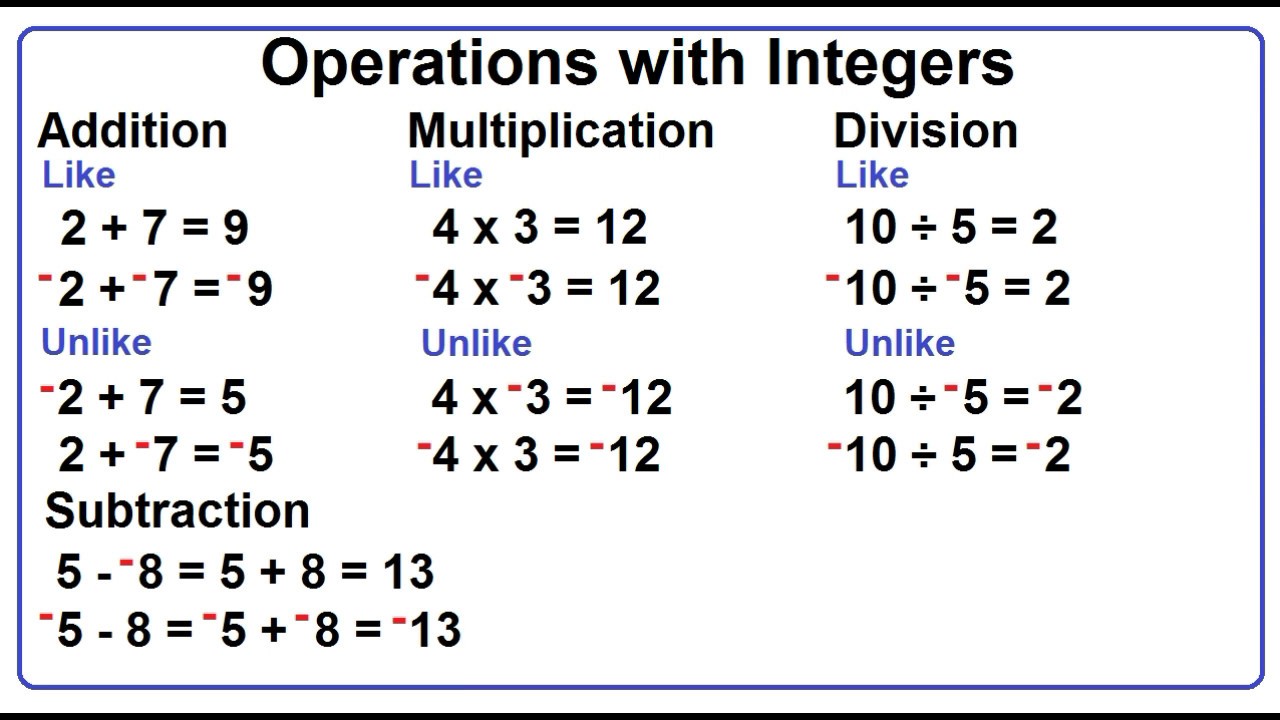
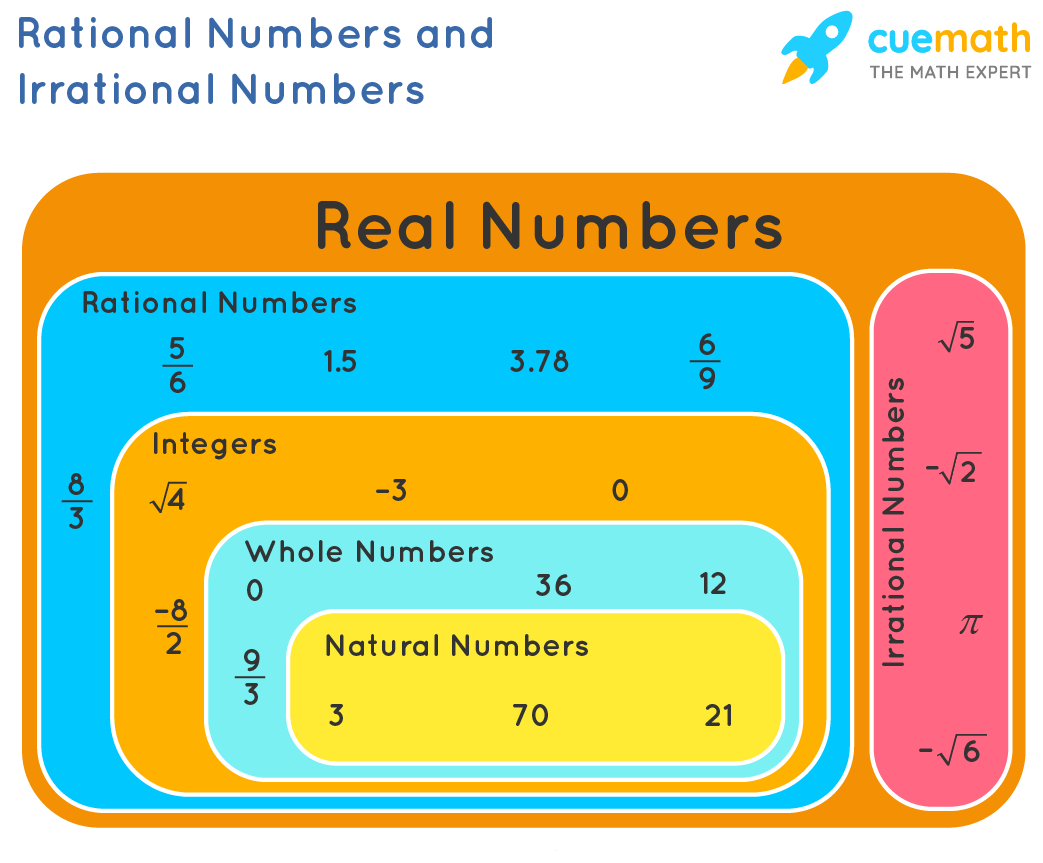
We can also use this definition to find some examples of rational numbers. It is worth noting that a number cannot be rational and irrational at the same time. We can then recall that the set of natural numbers is a subset of the integers,Īlthough it is beyond the scope of this explainer to prove this result, some numbers such as √ 2 or 𝜋 are not rationalĪnd are called irrational. This means that the set of integers isĪ subset of the set of rational numbers. First, we can note that all integers are rational numbers, Using this definition, we can see some interesting properties of the set of rational numbers. All natural numbers, whole numbers, and integers are rationals, but not all rational numbers are natural. Of the form 𝑎 𝑏 where 𝑎 and 𝑏 are integers and 𝑏 is nonzero. Integers and Rationals: Classification of Numbers. Every rational number can be written as a.
All integers are rational numbers plus#
The set of rational numbers, written ℚ, is the set of all quotients of integers. The rational numbers include all the integers, plus all fractions, or terminating decimals and repeating decimals.


 0 kommentar(er)
0 kommentar(er)
